This blog will explore the ways in which problem analysis of student mathematics difficulties can be applied within a problem-solving approach.
We will review the core principles of a multi-tier system of support (MTSS) framework, identify the steps within a problem-solving approach, and explore the ways in which problem analysis helps to inform intervention development within the context of mathematics instruction.
Core Principles of an MTSS Framework
A variety of definitions of an MTSS framework exist within the field of education; however, several common principles are apparent and have helped to shape much of the work within this area.
The National Association of State Directors of Special Education (Batsche et al., 2006) define eight core principles that capture some of the most important aspects and core beliefs of an MTSS framework:
- We can effectively teach all children.
- Intervene early.
- Use a multi-tier model of service delivery.
- Use a problem-solving model to make decisions within a multi-tier model.
- Use scientific, research-based validated intervention and instruction to the extent available.
- Monitor student progress to inform instruction.
- Use data to make decisions.
- Use assessment for screening, diagnostics, and progress monitoring.
The fourth core principle refers to utilizing a problem-solving model to make decisions. More specifically, educators and administrators should use a clearly defined problem-solving process that guides their team in identifying the problem, analyzing the size and effect of the problem, developing a plan for intervention to address the problem, implementing the plan, and examining the effectiveness of the intervention plan.
5-Step Problem-Solving Approach
The problem-solving approach utilized by the FastBridge Learning® system includes the following five steps:
- Problem identification
- Problem analysis
- Plan development
- Plan implementation
- Plan evaluation
Following this 5-step problem-solving approach helps to guide school teams of educators and administrators in engaging in data-based decision making: a core principle of the MTSS framework.
Problem identification
The first step in this problem-solving approach is problem identification. Christ and Arañas (2014) define a problem as a discrepancy between observed and expected performance. Regularly scheduled universal screening plays an important role in problem identification.
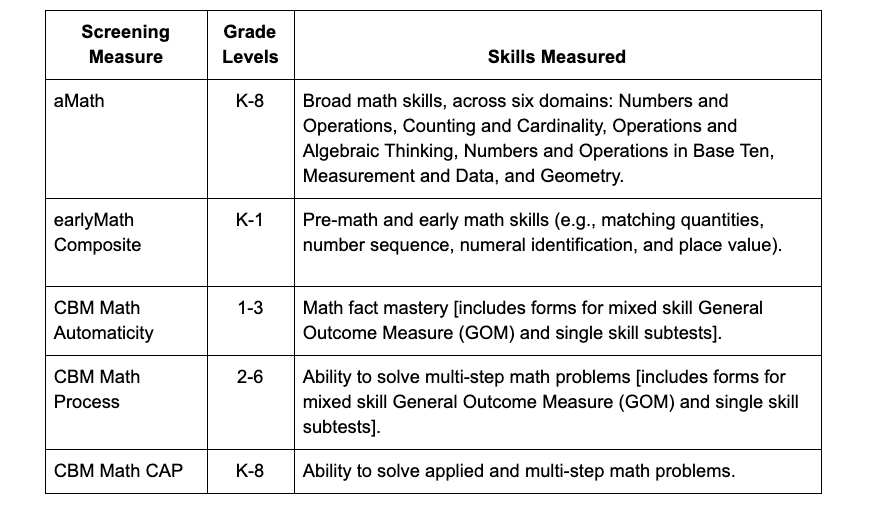
The FastBridge Learning® system offers a variety of screening measures for mathematics, which are summarized in the table below. While the results of regularly scheduled universal screening help to inform whether or not a discrepancy between observed and expected performance exists, problem analysis aims to identify the size and effects of the problem.
Table 1
Problem Analysis
After a problem has been identified, the problem must be defined through the method of problem analysis. Only after a problem has been sufficiently analyzed and defined, can the significance of a problem be understood (Brown-Chidsey & Bickford, 2016). Problem analysis can occur at both the individual level and the group level.
Problem Analysis at the Individual Level
At the individual level, a discrepancy between observed and expected performance may appear within an individual’s universal screening results. The FastBridge Individual Skills Reports can provide detailed information to support problem analysis.
The Individual Skills Report provides a snapshot of a given student’s risk, relative to benchmark goals, and also provides detailed results on an item-by-item basis. This item-by-item analysis provides insight into the skills the student demonstrates and which skills may require additional instructional support.
If the student’s performance is relatively close to the benchmark goal, the problem is likely to be understood as a minor problem. Item-by-item analysis can help a teacher determine if targeted reteaching of specific content may help the student to reach the goal, or if more intensive intervention may be necessary.
An example of a FastBridge Individual Skills Report for the earlyMath subtest Numeral Identification (Kindergarten) may be seen below.
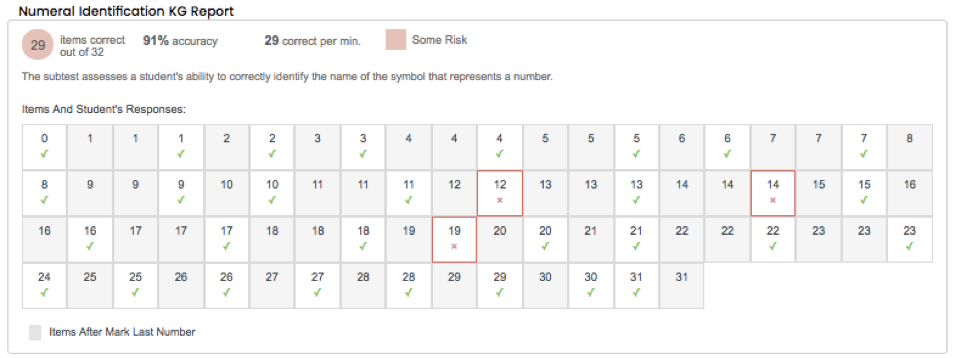
The above report suggests that the sample student is at “some risk” for difficulty with numeral identification. It also reveals that the student was able to identify the given numerals with 91% accuracy.
Additionally, the item-by-item analysis indicates that the student struggled to identify the following numerals: 12, 14, and 19. In this case, problem analysis may suggest that this is a minor problem, which may be remedied through targeted reteaching of the numerals 12, 14, and 19.
In contrast, if during problem analysis, an Individual Skills Report for Numeral Identification indicated very low accuracy and a substantial number of misidentified numerals, the results would suggest a more significant problem. Significant problems warrant planning for more intensive intervention.
Problem analysis at the group level
At the group level, problem analysis seeks to determine the size and effects of a problem at the class, grade, or school-wide level. FAST Group Screening Reports and Group Skills Reports help to provide insight about groups of students at risk for learning difficulties.
An example of a Group Screening Report for earlyMath may be seen below.
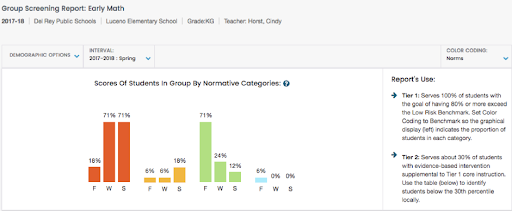
The Group Screening Report pictured above indicates that approximately 77% of Ms. Horst’s kindergarten class scored at or above the benchmark goal on the earlyMath Composite during the fall benchmark period, while 6% and 18% were identified as being at “some risk” and “high risk” respectively.
All students should demonstrate growth in math achievement throughout the school year. If there are students who met the fall benchmark for mathematics, but not the winter and/or spring benchmarks, problem analysis must occur.
In Ms. Horst’s class, we can see by the spring benchmark period, only 12% of her kindergarten class scored at or above the benchmark goal on the earlyMath composite, while 18% and 71% were identified as being at “some risk” and “high risk” respectively.
Problem analysis at the group level may aim to answer questions such as the following:
- What type of mathematics instruction was provided for these students?
- Did other kindergarten classrooms within the school experience a similar pattern of mathematics difficulty?
- With which specific mathematics skills (e.g., decomposing, number sequencing, numeral identification) did the group demonstrate success and difficulty?
- How close to the end-of-year mathematics goals are the students now?
In order to complete a thorough problem analysis, supplemental data about student performance and instructional practices may need to be collected.
For instance, classroom observations and/or a teacher interview would provide insight into the first question. Group Screening Reports for the other kindergarten classrooms in the school could help identify if this is a schoolwide pattern of difficulty or a classroom-specific pattern of difficulty.
An analysis of the Group Skills Report for Ms. Horst’s class would provide insight into students’ strengths and weaknesses across the earlyMath Composite’s subtests. Additional, targeted, follow-up assessment using selected earlyMath subtests could help to gather information about the final question.
The goal of problem analysis is to determine the significance of a problem and to develop a hypothesis about why a problem has occurred. The information gathered during the problem analysis stage is then used to inform the third step of the 5-step problem-solving approach: plan development.
References
Batsche, G., Elliott, J., Graden, J. L., Grimes, J., Kovaleski, J. F., Prasse, D., Schrag, J., & Tilly, W.D. (2006). Response to intervention: Policy considerations and implementation. Alexandria, VA: National Association of State Directors of Special Education, Inc.
Brown-Chidsey, R. and Bickford, R. (2016). Practical handbook of multi-tiered systems of support: Building academic and behavioral success in schools. New York, NY: The Guilford Press.
Christ, T.J., & Arañas, Y.A. (2014). Best practices in problem analysis. In A. Thomas & J. Grimes (Eds.), Best Practices in School Psychology VI. Bethesda, MD: National Association of School Psychologists.








